Consecutive Angles of a Parallelogram are Supplementary This shows that the consecutive angles are supplementary. = 2(∠A + ∠B) = 360º (We can substitute ∠C with ∠A and ∠D with ∠B since it is given that ∠A =∠C and ∠B =∠D) The sum of all the four angles of this quadrilateral is equal to 360°. Given: ∠A =∠C and ∠B=∠D in the quadrilateral ABCD.
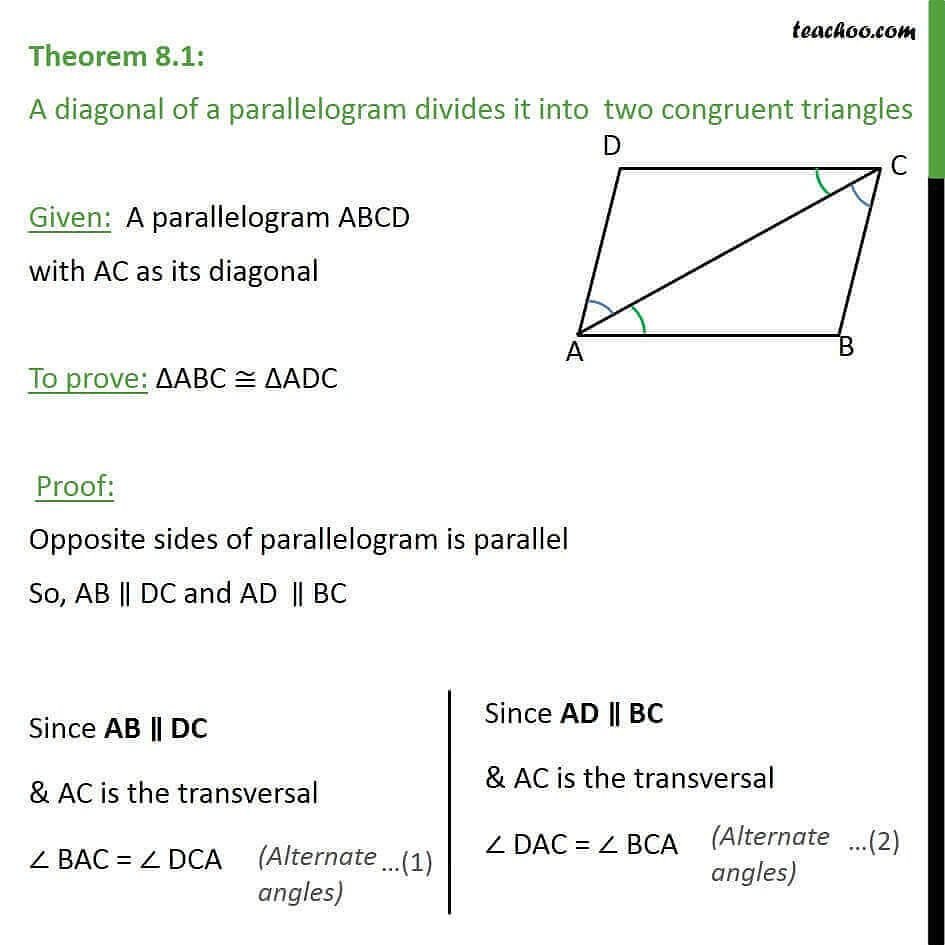
The converse of the above theorem says if the opposite angles of a quadrilateral are equal, then it is a parallelogram. Hence proved, that opposite angles in any parallelogram are equal. This gives ∠B = ∠D by CPCT (corresponding parts of congruent triangles). Thus, the two triangles are congruent, △ABC ≅ △ADC Proof: In the parallelogram ABCD, diagonal AC is dividing the parallelogram into two triangles. Given: ABCD is a parallelogram, with four angles ∠A, ∠B, ∠C, ∠D respectively. Theorem: In a parallelogram, the opposite angles are equal. Opposite Angles of a Parallelogram are Equal Let us learn about these two special theorems of a parallelogram in detail.
Consecutive angles of a parallelogram are supplementary.The opposite angles of a parallelogram are equal.
Two of the important theorems are given below: The theorems related to the angles of a parallelogram are helpful to solve the problems related to a parallelogram. Theorems Related to Angles of a Parallelogram